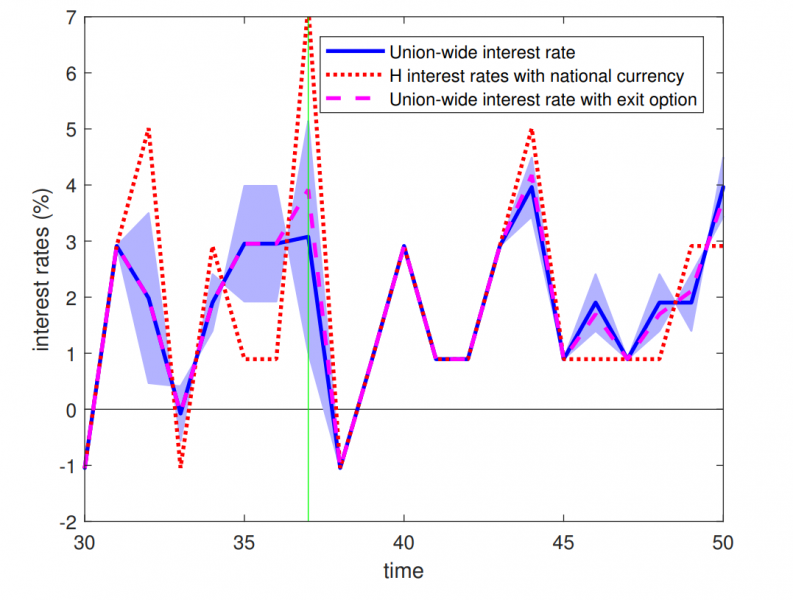
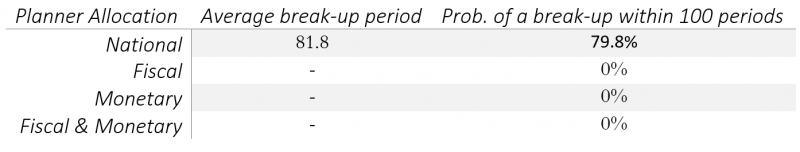
References
Alesina, A., and R. J. Barro. 2002. “Currency Unions.” The Quarterly Journal of Economics 409-436.
Corsetti, G., and P. Pesenti. 2005. “International Dimensions of Optimal Monetary Policy.” Journal of Monetary Economics 281-305.
Dmitriev, M., and J. Hoddenbagh. 2019. “Optimal fiscal transfers in a monetary union.” Journal of International Economics 91-108.
Fuchs, W., and F. Lippi. 2006. “Monetary Union with Voluntary Participation.” Review of Economic Studies 437-457.
Schmitt-Grohé, Stephanie, and Martin Uribe. 2016. “Dowanward Nominal Wage Rigidity, Currency Pegs and Involuntary Unemployment.” Journal of Political Economy 1466-1514.