SUERF

With boundedly rational agents, an inflation-targeting rule for monetary policy can yield higher economic welfare than history-dependent rules like price-level targeting (PLT) or average-inflation targeting (AIT). In a New Keynesian model with sticky prices and wages, bounded rationality attenuates the stabilising effects arising from the “expectations channel” that typically favour history-dependent rules. For demand shocks, such rules lose their advantage vis-à-vis inflation targeting even when considering the effective lower bound. For supply shocks that induce a trade-off between stabilising inflation and economic activity, an inflation-targeting rule even outperforms history-dependent rules for a sufficiently high degree of bounded rationality. An exponential average inflation-targeting rule, which features a variable degree of history dependence, performs remarkably well independently of the degree of bounded rationality.

1. History-dependent rules and the expectations channel

The long-term decline in the natural real rate together with an effective lower bound (ELB) on nominal interest rates limits the space for monetary policy to provide economic stimulus when inflation rates are too low.1 Consequently, major central banks have analysed several alternative interest-rate rules within their reviews of their monetary policy strategy to evaluate possibilities of providing policy stimulus at the ELB.2 More recently, monetary policy has also been challenged by severe supply shocks that induce a trade-off between stabilizing inflation rates and real activity.

In principle, history-dependent strategies like average-inflation targeting (AIT) or price-level targeting (PLT) can help with both challenges via the expectations channel. History-dependent strategies promise to “make up” for past and current deviations of prices or inflation from their respective target in the future. When inflation is below target during an ELB episode, a history-dependent strategy keeps the policy rate low even after the ELB ceases to bind in order to compensate for the inflation shortfall through a future expansion. If agents rationally expect higher inflation in the future, real rates will already be lowered today, thereby stabilising contemporaneous inflation as well.3 In case of an adverse supply shock that increases inflation and decreases economic activity, a history-dependent strategy can mitigate the arising trade-off by promising to compensate high contemporaneous inflation rates through lower future rates. Lower expected future inflation rates imply a higher real rate and hence a lower contemporaneous inflation rate – without having to slow down real activity further.

The efficacy of history-dependent strategies therefore crucially depends on agents’ expectations being rational and forward-looking. This is true for both supply shocks that induce economic trade-offs and demand shocks that drive the policy rate to the ELB. However, recent research on expectation formation has increasingly documented substantial deviations from full information rational expectations.4 Thus, a key question for monetary policy makers arises whether history-dependent strategies still perform well despite these deviations.

2. Comparison of optimised interest-rate rules

In Dobrew, Gerke, Kienzler and Schwemmer (2023), we analyse the performance of different optimised history-dependent interest-rate rules, comparing them to an optimised non-history dependent inflation-targeting (IT) rule. Our model economy with boundedly rational agents is occasionally constrained by an ELB and has a meaningful role for supply shocks. To that end, we employ a New Keynesian model with sticky prices and sticky wages as in Erceg, Henderson and Levin (2000). In this set-up, technology shocks induce a trade-off between stabilising inflation and real activity. In the spirit of Gabaix (2000), we assume that the agents are partially myopic in the sense that they discount expectations of future variables. Myopia weakens the stabilising effects of the expectations channel, both at the ELB and in case of trade-offs arising from supply shocks.

To compare the performance of the different monetary policy rules under different degrees of myopia we proceed as follows: For each monetary policy rule and each degree of myopia, we span a wide grid over the parameters of the policy rule. At each grid node we run a stochastic simulation within which we solve the model with the extended path algorithm.5 From the generated time series, we calculate the means and variances of the model variables. An advantage of our model structure is that we can use the model-consistent welfare loss function to evaluate the performance of each rule.6 Thus, for each degree of myopia and conditional on the type of shock, we can compare the welfare performance across different types of optimised interest-rate rules.7

The interest-rate rules we consider are an IT rule, an AIT(32) rule with an averaging window of 32 quarters, an AIT(16) rule with an averaging window of 16 quarters, a PLT rule and two exponential AIT rules (eAIT), which are further explained below.

3. Performance of different rules under demand and supply shocks

Figure 1 shows the welfare loss (vertical axis) of different monetary policy rules for different degrees of myopia (horizontal axis) under demand shocks. A myopia parameter of one corresponds to rational expectations (right corner). Smaller myopia parameters, i.e. higher degrees of myopia, imply that agents are less rational and more bounded in their expectations (moving to the left). The solid blue line represents the non-history-dependent IT rule while other lines represent different history-dependent interest-rate rules.

Figure 1: Welfare comparison of different monetary policy rules for demand shocks

For demand shocks history-dependent interest-rate rules lose their advantage vis-à-vis the IT rule as the degree of myopia increases and the strength of the expectations channel fades. Since there is no trade-off for demand shocks but only a friction arising from the ELB there is virtually no difference anymore between the optimised history-dependent rules and the optimised IT rule even for moderate degrees of myopia.

For supply shocks that induce economic trade-offs the IT rule even outperforms history-dependent rules for moderate to high degrees of myopia, as shown in Figure 2. This result arises because history-dependent rules induce a lot of volatility in real activity in order to stabilise inflation when the expectations channel is impaired.

Figure 2: Welfare comparison of different monetary policy rules for supply shocks

4. Exponential AIT as a robust interest-rate rule

In practice, monetary policy makers face considerable uncertainty about the degree of myopia. A robust interest-rate rule should therefore perform well across different degrees of myopia and independently of the type of shock occurring. Ideally, on the one hand, such a rule should exhibit some form of history-dependence in order to reap the benefits of this feature in case the degree of myopia is low. On the other hand, it should resemble an IT rule when the degree of myopia is high.

In principle, an AIT rule can fulfil these requirements as its performance is between the IT and the PLT rule for both low and high degrees of myopia. However, and especially for trade-off inducing technology shocks, conventional AIT that features an arithmetic, or simple, moving average of inflation rates exhibits an inherent volatility-inducing character. The reason is that any past deviation of inflation from its target directly affects the inflation average for a given time frame. This calls for monetary policy to move inflation in the opposite direction in order to achieve the inflation target on average. But once the initial deviation drops out of the averaging window, monetary policy needs to now move inflation in the opposite direction again (i.e., the direction inflation had originally deviated) to achieve its inflation target on average. As a result, inflation would cycle between under- and overshooting its target. This cyclical behaviour typically fades only slowly over time. Conventional AIT thus has the disadvantage that it induces periodic fluctuations in the inflation rate (and the output gap), thus adding intrinsic volatility and lowering welfare.

A possibility to circumvent this disadvantage while retaining the advantages of the conventional AIT rule is to employ an eAIT rule. Under an eAIT rule, monetary policy responds to an exponential moving average of the current and all past inflation deviations instead of an arithmetic, or simple, moving average with a fixed averaging window as in conventional AIT rules.8 This has two crucial consequences, one general and one specific to our setting with bounded rationality.

As a general implication of an eAIT rule past inflation rates never actually fully drop out of the average, avoiding the inherent volatility-inducing character of the AIT rule. Instead, past inflation rates receive an exponentially decaying weight over time, implying that deviations closer to the present period receive a higher weight and more attention when calculating average inflation. This “tilts” the character of the eAIT rule towards an IT rule and directly contrasts with a conventional AIT rule, where all (past) inflation deviations entering the average receive equal weights. Specifically, the effect of this “tilting” is more pronounced for higher degrees of bounded rationality as this further reduces the influence that past inflation rates have on expectations about future inflation rates.

Consequently, as Figure 2 shows, the eAIT rule performs remarkably well under supply shocks, independently of the degree of myopia. On the one hand, it preserves the history-dependent character of the conventional AIT rule and thus approximates the superior welfare performance of a PLT rule for low degrees of myopia. On the other hand, the eAIT rule shifts weights in the targeted average to the present and is thus able to approximate the performance of an IT rule when the degree of myopia is high. Moreover, across all degrees of myopia the eAIT rule avoids inherently inducing volatility like the AIT rule.

References

Adjemian, S., Bastani, H., Juillard, M., Karamé, F., Maih, J., Mihoubi, F., Mutschler, W., Perendia, G., Pfeifer, J., Ratto, M. and Villemot, S. (2021): Dynare: Reference Manual Version 4, Dynare Working Papers 1, CEPREMAP.

Afrouzi, H., Kwon, S. K., Landier, A., Ma, Y. and Thesmar, D. (2023): Overreaction in Expectations: Evidence and Theory, The Quarterly Journal of Economics, 138, 1713–1764.

Brand, C., Bielecki, M. and Penalver, A. (2018): The Natural Rate of Interest: Estimates, Drivers, and Challenges to Monetary Policy, Occasional Paper Series 217, European Central Bank.

Coibion, O., Gorodnichenko, Y. and Kamdar, R. (2018): The Formation of Expectations, Inflation, and the Phillips Curve, Journal of Economic Literature, 56, 1447–1491.

Dobrew, M., Gerke, R., Kienzler, D. and Schwemmer, A. (2023): Monetary Policy Rules under Bounded Rationality, Deutsche Bundesbank Discussion Paper, No. 18/2023.

Erceg, C. J., Henderson, D. W. and Levin, A. T. (2000): Optimal Monetary Policy with Staggered Wage and Price Contracts, Journal of Monetary Economics, 46, 281–313.

Fair, R. and Taylor, J. B. (1983): Solution and Maximum Likelihood Estimation of Dynamic Nonlinear Rational Expectations Models, Econometrica, 51, 1169–85.

Gabaix, X. (2020): A Behavioral New Keynesian Model,” American Economic Review, 110, 2271–2327.

Holston, K., Laubach, T. and Williams, J. C. (2023): Measuring the Natural Rate of Interest after COVID-19, Federal Reserve Bank of New York Staff Reports, No. 1063.

Holston, K., Laubach, T. and Williams, J. C. (2017): “Measuring the Natural Rate of Interest: International Trends and Determinants,” Journal of International Economics, 108, 59–75.

^1.
See e.g. Holston, Laubach and Williams (2023), Holston, Laubach and Williams (2017) or Brand, Bielecki and Penalver (2018).
^2.
E.g. the Federal Reserve released a revised Statement on Longer-Run Goals and Monetary Policy Strategy in 2020. The Eurosystem concluded its strategy review in 2021. The Bank of Canada renewed its flexible inflation-targeting framework in 2021. The Bank of Japan has conducted a smaller policy review in the beginning of 2021.
^3.
Other possibilities to mitigate the ELB constraint in the realm of monetary policy include negative interest rates, forward guidance, and asset purchase programmes.
^4.
For survey evidence, see e.g. Coibion, Gorodnichenko and Kamdar (2018). For experimental evidence, see e.g. Afrouzi, Kwon, Landier, Ma and Thesmar (2023).
^5.
The extended path algorithm is described in Fair and Taylor (1983). We implement the computations with the software package Dynare, see Adjemian, Bastani, Juillard, Karamé, Maih, Mihoubi, Mutschler, Perendia, Pfeifer, Ratto and Villemot (2021).
^6.
The arguments in the loss function are the volatility of price inflation, wage inflation, and the output gap.
^7.
We consider a demand shock in the form of a discount factor shock and a supply shock in the form of a technology shock.
^8.
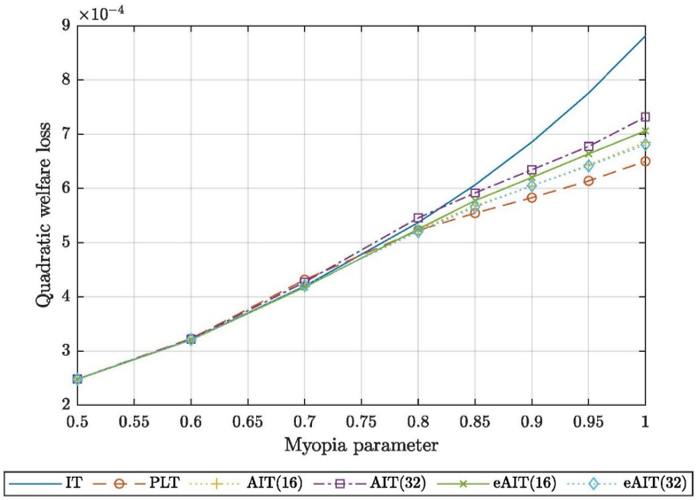
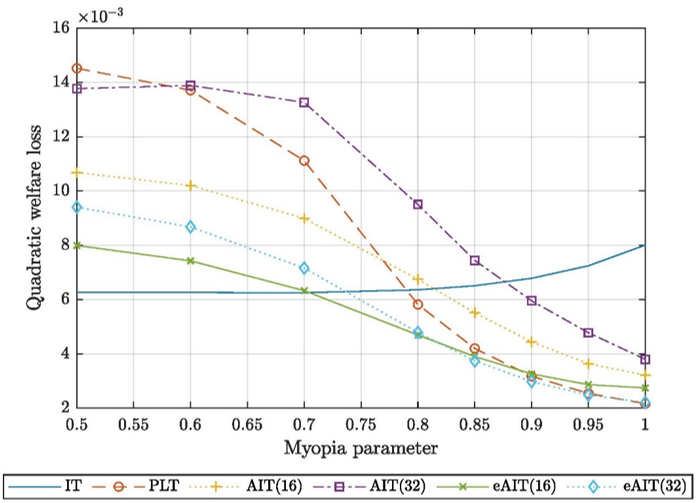
eAIT(32) in the figures represents an eAIT rule with a relatively high exponential smoothing parameter, while eAIT(16) represents an eAIT rule with a lower exponential smoothing parameter. The numbers are, however, not comparable to the averaging windows in the conventional AIT rules.

Monetary policy rules under bounded rationality

Author(s):

Keywords:

Download:

JEL Codes:

1. History-dependent rules and the expectations channel

2. Comparison of optimised interest-rate rules

3. Performance of different rules under demand and supply shocks

4. Exponential AIT as a robust interest-rate rule

References

About the authors

More on these topics

Monetary policy rules under bounded rationality

Author(s):

Keywords:

Download:

JEL Codes:

1. History-dependent rules and the expectations channel

2. Comparison of optimised interest-rate rules

3. Performance of different rules under demand and supply shocks

4. Exponential AIT as a robust interest-rate rule

References

About the authors

More on these topics

More on these topics

The 2021-2022 inflation surges and the monetary policy response throu...

Philip R. Lane

It ain't over yet ˗ It takes time to go back to the old regime of in...

Juhana Hukkinen, Matti Viren

Geopolitical Risks and Their Impact on Global Macro-Financial Stabili...

Martin Hodula, Jan Janku, Simona Malovana, Ngoc Anh Ngo

Energy shocks, inflation and the ECB’s monetary policy

Stefano Neri

The macroeconomic effects of liquidity supply during financial crises

Davide Porcellacchia, Kevin Sheedy

Whither inflation targeting as a global monetary standard?

Claudio Borio